What Scale Factor Takes the Original Polygon

Scale Factor

Scale factor is a number by which the size of any geometrical figure or shape can be changed with respect to its original size. It is used to draw the increased or reduced shape of any given figure. It should be noted that the scale factor helps in changing the size of the figure and not its shape.

For example, a rectangle has a length of 5 units and a width of 2 units. Now, if we increase the size of this rectangle by a scale factor of 2, the sides will become 10 units and 4 units, respectively. Let us learn how to find the scale factor and how to use the scale factor to get the dimensions of the changed figures.

1.	How to Find the Scale Factor?
2.	How to Identify the Scale Factor?
3.	Solved Examples on Scale Factor
4.	Practice Questions on Scale Factor
5.	FAQs on Scale Factor

How to Find the Scale Factor?

We know that the scale factor is used to increase or decrease the size of an object. It can be calculated if the dimensions of the original figure and the dimensions of the dilated (increased or decreased) figure are known.

Scale Factor Formulas

The basic formula to find the scale factor of a figure is: Scale factor = Dimensions of the new shape ÷ Dimensions of the original shape.

This can also be used to calculate the dimensions of the new figure or the original figure by simply substituting the values in the same formula.

Observe the following image which shows how the scale factor can change the original figure to its larger and smaller versions. In the following figure, the original rectangle has the dimensions given as 3 units and 2 units. To create an expanded image, the dimensions are multiplied by the scale factor of 3 using the formula: Dimensions of the new shape = Dimensions of the original shape × Scale factor. This gives the new dimensions as 9 units and 6 units respectively. Similarly, to create a reduced image, we multiply the original dimensions with the scale factor of 1/2. This gives the reduced dimensions as 1.5 units and 1 unit.

How to Identify the Scale Factor?

When the size of an image is increased, we say that it has been scaled up and when it is decreased, we say that it has scaled down.

Scale Up - This means that a smaller figure is enlarged to a bigger one. In this case, the scale factor can be calculated by a formula, which is another version of the basic formula given in the previous section. Scale factor: Larger figure dimensions ÷ Smaller figure dimensions. The scale factor for scaling up is always greater than 1. For example, if the dimension of the larger figure is 15 and that of the smaller one is 5, let us place this in the formula which makes it: 15 ÷ 5 = 3. Thus, we can see that the scale factor is greater than 1.

Scale Down - This means that a larger figure is reduced to a smaller one. Even in this case, the scale factor can be calculated by a formula, which is another version of the same formula given in the previous section. Scale factor: Smaller figure dimensions ÷ Larger figure dimensions. The scale factor for scaling down is always less than 1. For example, if the dimension of the smaller figure is 8 and that of the larger one is 24, let us place this in the formula which makes it: 8 ÷ 24 = 1/3. Thus, we can see that the scale factor is less than 1.

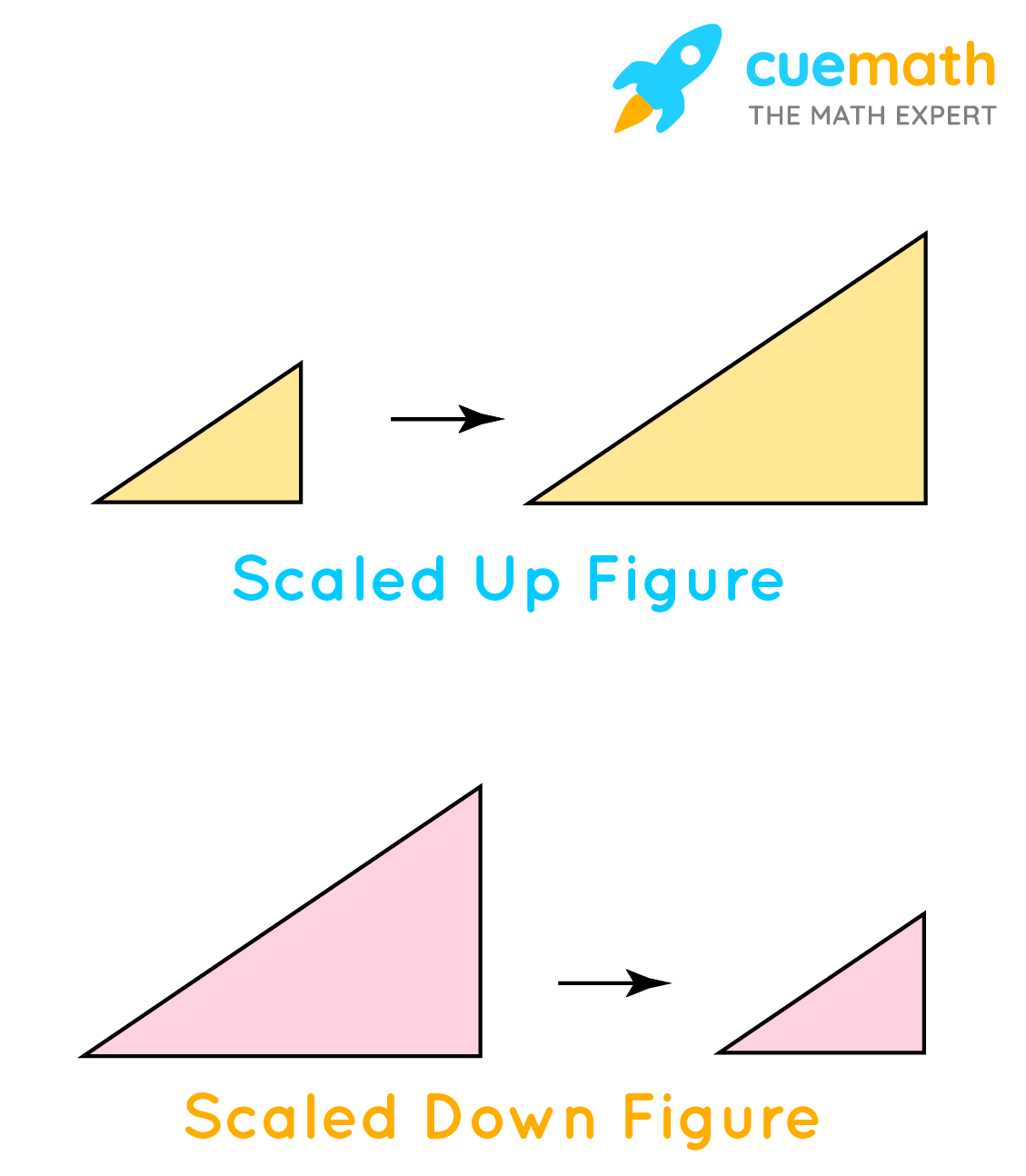
Observe the following figures which show a scaled up figure and a scaled down figure.

Important Notes

The following points should be remembered while studying about the scale factor:

• The scale factor of a dilated figure is denoted by 'r' or 'k'.
• If the scale factor is more than 1 (k > 1), the image is enlarged.
• If the scale factor is less than 1 (0< k <1), the image is contracted.
• If the scale factor is 1 (k = 1), the image remains the same.
• The scale factor cannot be zero.

Related Topics on Scale Factor

Check out the articles mentioned below to learn more about scale factor.

• Scale
• Dilation Geometry

1. Example 1. Write the correct scale factor formulas for the following situations.
   a) If the image has to be enlarged.
   b) If image has to be reduced.

   Solution:

   a) If the image has to be enlarged, the scale factor is more than 1 (k > 1). This means the image is scaled up.
   The scale factor formula = Larger figure dimensions ÷ Smaller figure dimensions

   b) If the image needs to be reduced, the scale factor is less than 1 (0<k<1). This means the image is scaled down.
   The scale factor formula = Smaller figure dimensions ÷ Larger figure dimensions

2. Example 2. There are two similar polygons as shown below. Find the scale factor used to create the smaller polygon.

   

   Solution:The given dimension of the bigger polygon is 40 units. The given dimension of the smaller polygon is 20 units. Here, the figure is scaled down.
   The scale factor = Smaller figure dimensions ÷ Larger figure dimensions = 20/40 = 1/2.
   Therefore, the scale factor used to create the smaller polygon is 1/2.

3. Example 3. A triangle was increased by a scale factor of 2 resulting in the new dimensions as 6 units by 10 units by 12 units. Find the dimensions of the original triangle.

   Solution:

   The new dimensions of the triangle are: 6 units; 10 units; and 12 units; The scale factor = 2

   Scale factor = Dimensions of the new shape ÷ Dimensions of the original shape. Substituting the values in the formula for all the three dimensions:

   a.) 2 = 6 ÷ Dimensions of the original shape

   Dimensions of the original shape = 6 ÷ 2 = 3 units

   b.) 2 = 10 ÷ Dimensions of the original shape

   Dimensions of the original shape = 10 ÷ 2 = 5 units

   c.) 2 = 12 ÷ Dimensions of the original shape

   Dimensions of the original shape = 12 ÷ 2 = 6 units
   Therefore, the original dimensions of a triangle were = 3 units, 5 units, and 6 units

go to slidego to slidego to slide

Great learning in high school using simple cues

Indulging in rote learning, you are likely to forget concepts. With Cuemath, you will learn visually and be surprised by the outcomes.

Book a Free Trial Class

FAQs on Scale Factor

What is a Scale Factor?

Scale factor is used to draw the increased or reduced shape of any given figure. It is a number by which the size of any geometrical figure or shape can be changed with respect to its original size. It helps in changing the size of the figure but not its shape.

What Happens if the Scale Factor is Greater Than 1?

If the scale factor is more than 1 (k > 1), it means that the given figure needs to be enlarged.

What Does a Scale Factor of 0.5 Mean?

A scale factor of 0.5 means that the changed image will be scaled down. For example, the original figure of a square has one of its sides as 6 units. Now, let us use the scale factor of 0.5, to change its size. Using the formula:Dimensions of the new shape = Dimensions of the original shape × Scale factor. Substituting the values in the formula: the dimensions of the new square will be = 6 × 0.5 = 3 units. This shows that a scale factor of 0.5 changed the figure to a smaller one.

How do you Find the Scale Factor?

The basic formula to find the scale factor of a figure is: Scale factor = Dimension of the new shape ÷ Dimension of the original shape.

What Happens if the Scale Factor is Less Than 1?

If the scale factor is less than 1 (0< k <1), then the new image that is formed will be contracted or scaled down. In other words, the new figure will have smaller dimensions as compared to the original figure after it is resized using the scale factor which is less than 1.

Where do we Use a Scale Factor?

The scale factor is a number by which the size of any geometrical figure or shape can be changed with respect to its orginal size. When things are too large, we use scale factors to calculate smaller, proportional measurements. It is used to compare two similar geometric figures and also in other fields like cooking, where the ingredients can be reduced or increased according to the given situation.

What Scale Factor Takes the Original Polygon

Source: https://www.cuemath.com/geometry/scale-factor/

Share this post